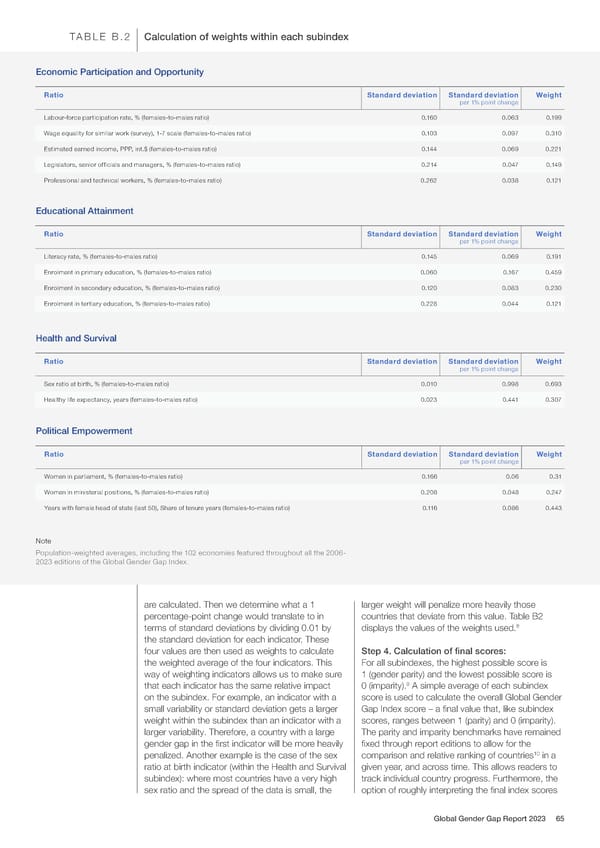
TABLE B.2 Calculation of weights within each subindex Economic Participation and Opportunity Ratio Standard deviation Standard deviation Weight per 1% point change Labour-force participation rate, % (females-to-males ratio) 0.160 0.063 0.199 Wage equality for similar work (survey), 1-7 scale (females-to-males ratio) 0.103 0.097 0.310 Estimated earned income, PPP, int.$ (females-to-males ratio) 0.144 0.069 0.221 Legislators, senior officials and managers, % (females-to-males ratio) 0.214 0.047 0.149 Professional and technical workers, % (females-to-males ratio) 0.262 0.038 0.121 Educational Attainment Ratio Standard deviation Standard deviation Weight per 1% point change Literacy rate, % (females-to-males ratio) 0.145 0.069 0.191 Enrolment in primary education, % (females-to-males ratio) 0.060 0.167 0.459 Enrolment in secondary education, % (females-to-males ratio) 0.120 0.083 0.230 Enrolment in tertiary education, % (females-to-males ratio) 0.228 0.044 0.121 Health and Survival Ratio Standard deviation Standard deviation Weight per 1% point change Sex ratio at birth, % (females-to-males ratio) 0.010 0.998 0.693 Healthy life expectancy, years (females-to-males ratio) 0.023 0.441 0.307 Political Empowerment Ratio Standard deviation Standard deviation Weight per 1% point change Women in parliament, % (females-to-males ratio) 0.166 0.06 0.31 Women in ministerial positions, % (females-to-males ratio) 0.208 0.048 0.247 Years with female head of state (last 50), Share of tenure years (females-to-males ratio) 0.116 0.086 0.443 Note Population-weighted averages, including the 102 economies featured throughout all the 2006- 2023 editions of the Global Gender Gap Index. are calculated. Then we determine what a 1 larger weight will penalize more heavily those percentage-point change would translate to in countries that deviate from this value. Table B2 8 terms of standard deviations by dividing 0.01 by displays the values of the weights used. the standard deviation for each indicator. These four values are then used as weights to calculate Step 4. Calculation of 昀椀nal scores: the weighted average of the four indicators. This For all subindexes, the highest possible score is way of weighting indicators allows us to make sure 1 (gender parity) and the lowest possible score is 9 that each indicator has the same relative impact 0 (imparity). A simple average of each subindex on the subindex. For example, an indicator with a score is used to calculate the overall Global Gender small variability or standard deviation gets a larger Gap Index score – a 昀椀nal value that, like subindex weight within the subindex than an indicator with a scores, ranges between 1 (parity) and 0 (imparity). larger variability. Therefore, a country with a large The parity and imparity benchmarks have remained gender gap in the 昀椀rst indicator will be more heavily 昀椀xed through report editions to allow for the 10 penalized. Another example is the case of the sex comparison and relative ranking of countries in a ratio at birth indicator (within the Health and Survival given year, and across time. This allows readers to subindex): where most countries have a very high track individual country progress. Furthermore, the sex ratio and the spread of the data is small, the option of roughly interpreting the 昀椀nal index scores Global Gender Gap Report 2023 65
 Global Gender Gap Report 2023 Page 64 Page 66
Global Gender Gap Report 2023 Page 64 Page 66